General Angles and Standard Position

The precise definition of an angle is the union of two rays with a common vertex.  When we measure angles in degrees, we place a circle at the vertex and count the number of marks out of 360 on the circle that are interior to the angle.  This always gives us a number between 0 and 180, and that is what we might call the "protractor measure" of the angle.

There are applications, such as rotating wheels, where it is convenient to expand our definition of angle so measures can be any number of degrees, including positive and negative values.  Such a definition is given as follows:

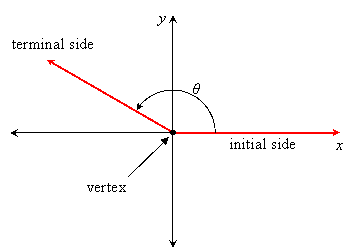
Fix one ray, called the initial side, and place a copy of this ray on top of itself.  Then rotate the new ray, called the terminal side, about its vertex any specified number of degrees (marks on a circle which contains 360 marks and whose center is at the vertex) counterclockwise, or clockwise if the degrees are indicated by a negative number.  When the initial side is place along the positive x-axis with its vertex at the origin, the angle is said to be in standard position:

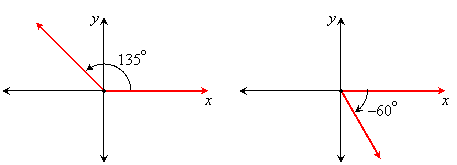
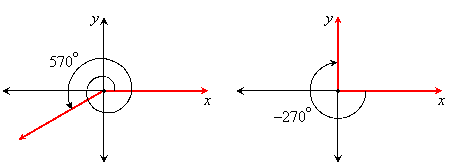
Here are some examples of angles drawn in standard position:

  

 

toc | return to top | next page