Side-Angle-Angle Theorem

The fourth result (theorem) follows from ASA and the fact that the angles of a triangle add to  (which will be proved later using the parallel postulate):

 

SAA

If two angles of one triangle are congruent to two angles of another and a side of the first triangle that is not common to both angles is congruent to a side of the second triangle that is not common to both angles, then the triangles are congruent:

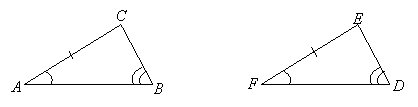
If , , and , it follows by SAA that .

 

toc | return to top | previous page | next page