By repeated applications of the Triangle Midsegment Theorem, we can arrive at more general results:

The Proportional Segments Theorem:

Three or more parallel lines cut any two transversals into proportional segments.

For example, in the following figure, :

Sample Problem:  Find x:

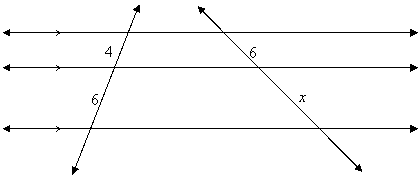
Solution:     Set up the proportion:  .  Then cross-multiply and solve:

                    , so

Corollary:   

If a segment with endpoints on two sides of a triangle is parallel to the third side, it divides the two sides into proportional segments.

Sample Problem:  Find x:

Solution:         This is the same as the last problem, as can by seen by drawing a third parallel line at the top vertex of the triangle:

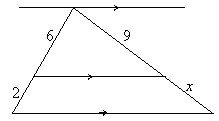
Therefore, it has the same solution:  , so x = 9.