, N is on 
, and 
and 
Parallel Lines and Proportional Segments
The Triangle Midsegment Theorem
If you draw any triangle, locate the midpoints of two sides, and draw a segment between these midpoints, it appears that this segment is parallel to the third side and half its length:
This result follows from a very important theorem, called the Triangle Midsegment Theorem, which also leads to results about similarity of figures. (Two figures are said to be similar if they have the same shape, but not necessarily the same size.)
Triangle Midsegment Theorem:
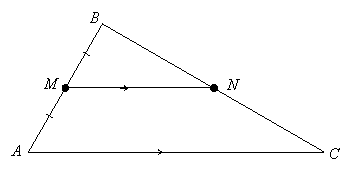
A segment joining two sides of a triangle, parallel to the third side, and containing the midpoint of one of the two sides also contains the midpoint of the other side, and is half the length of the parallel side.
| Given: | M is the midpoint of , N is on , and |
|
| Prove: | N is the midpoint of and |
|
Proof of the Triangle Midsegment Theorem
To shorten proofs in geometry, we can sometimes prove preliminary results. In the case of the Triangle Midsegment Theorem, a preliminary result is that opposite sides of a parallelogram are congruent. Recall that a parallelogram is a quadrilateral with opposite sides congruent. So first we will prove:
Theorem: The opposite sides of a parallelogram are congruent.
Proof:
|
Let the parallelogram be ABCD, and draw the diagonal
|
 |
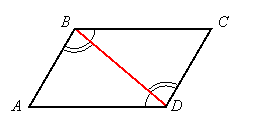Now we can prove the Triangle Midsegment Theorem:
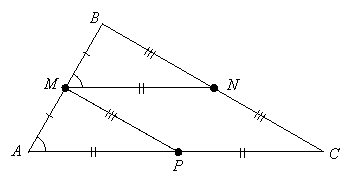
| Given: | M is the midpoint of  , N is on  , and  |
 |
| Prove: | N is the midpoint of  and  |
|
Proof:
We will show that the result follows by proving two triangles congruent. First locate point P on side 
so 
, and construct segment 
:
Notice that 
is a transversal for parallel segments 
and 
, so the corresponding angles, 
and 
are congruent:
Now, for 
and 
we have:
(because M is the midpoint of 
)
and 
Therefore, these triangles are congruent by the SAS postulate, and so their other corresponding parts are congruent: 
, 
, and 
. Also, since 
(this was given), 
because these are corresponding angles for the transversal 
. Therefore, 
. But these are corresponding angles for segments 
and 
with transversal 
, so by the Corresponding Angle Theorem, 
. Thus, MNCP is a parallelogram, and by Example 3 from the previous lesson, its opposite sides are equal:
and 
:
Since BN and NC are both equal to MP, they are equal to each other, so N is the midpoint of 
. Likewise, since AP and PC are both equal to MN, P is the
midpoint of 
: 
. But AP = MN. Therefore, 
.
Consequences of the Triangle Midsegment Theorem
From the Triangle Midsegment Theorem it follows that a segment joining the midpoints of two sides of a triangle is parallel to the third side and half its length, since there can be only one line through a given point (the midpoint of one side) parallel to another line (the third side).
Another way to state the Triangle Midsegment Theorem is:
If an angle is cut by two parallel lines so that the pairs of segments on one side of the angle are equal, then the pairs of segments on the other side of the angle will be equal and the segment on the parallel between the vertex of the angle and the other parallel is half as long as the segment on the other parallel:
This result can be repeated as in the following example:
Problem: Suppose parallel lines l, m, n, and p cut 
so that segments 
, 
, 
, and 
all have the same length:
If AP = 8 and EQ = 28, then how long are 
and 
?
Solution: Lines l and m cut the angle as in the Triangle Midsegment Theorem, so we know the following lengths, where for the time being we call BP x:
Lines m and p also cut the angle as in the Triangle Midsegment Theorem, so we know these lengths:
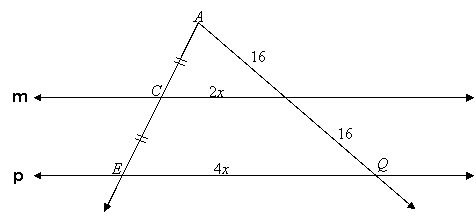
Now we can see that AQ = 32. Since P is between A and Q, AP + PQ = AQ, which tells us PQ = 24. Also, EQ = 27, and this is 4x, so x = 7: AP = 7.
The Proportional Segments Theorem
By repeated applications of the Triangle Midsegment Theorem, we can arrive at more general results:
The Proportional Segments Theorem:
Three or more parallel lines cut any two transversals into proportional segments.
For example, in the following figure, 
:
Sample Problem: Find x:
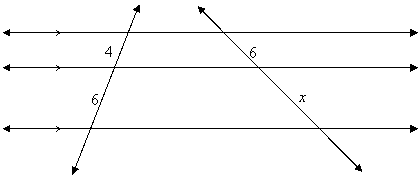
Solution: Set up the proportion: 
. Then cross-multiply and solve:
, so 
Corollary:
If a segment with endpoints on two sides of a triangle is parallel to the third side, it divides the two sides into proportional segments.
Sample Problem: Find x:
Solution: This is the same as the last problem, as can by seen by drawing a third parallel line at the top vertex of the triangle:
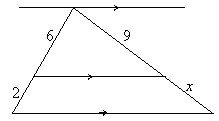
Therefore, it has the same solution: 
, so x = 9.
. Then since opposite sides are parallel (this is the definition of a parallelogram), 
and 
since these are alternate interior angles for the parallel sides with transversal 
. Thus 
by ASA since they have side 
in common. Therefore 
and 
since these are corresponding parts of the congruent triangles.