In Euclidean geometry, the sum of the angles of any triangle is 180^o.  If you extend one side of a triangle, you get an exterior angle that is supplementary to its adjacent, interior angle:

The other two interior angles are called remote interior angles since they are "remote" from the exterior angle, and since all three angles of the triangle add to 180^o, these remote interior angles add to the exterior angle.  In other words, we have:

The Exterior Angle Theorem

The measure of an exterior angle of a triangle is equal to the sum of the measures of the two remote interior angles. 

Proof:

Let the triangle be  and the exterior angle be :

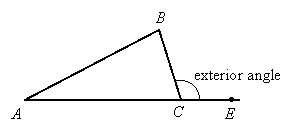
Then we need to prove that .  Since the exterior angle,  is supplementary to its adjacent interior angle, , we know , so .  But we also know the angles in the triangle add to 180^o:  , so .  Since  and  are both equal to , they are equal to each other by the transitive property:  .

Here is an obvious result that follows immediately:

Corollary to the Exterior Angle Theorem

The measure of an exterior angle is always greater than the measure of either remote interior angle.

Proof:

In the above setup, .  If we drop one of the measures on the right, we have  or  since angles always have positive measure.