Parallel Lines

Two lines are said to be parallel when they are contained in the same plane and do not intersect.  This is the definition.  That parallel lines exist is an assumption (postulate) of Euclidean geometry:

Parallel Postulate:  Given a line and a point not on that line, there is one and only one line through the given point parallel to the given line.

Parallel lines are like the rails of a train track, and you might think of defining them this way, as lines that are the same distance apart everywhere.  The problem with this kind of definition is it assumes both tracks are straight.  Though this seems an obvious possibility, when you go into the vast universe it is not that obvious.  Parallel lines puzzled the best mathematicians for centuries until it was realized that we must assume they exist (you can't prove they exist from simpler postulates).  The problem with parallel lines lies in the notion that the lines have infinite extent.

Euclid used a somewhat different parallel postulate in trying to avoid the notion of the infinite.  He observed that when two parallel lines are intersected by a third line, called a transversal, then if you measure two angles formed by these three lines, on the same side of the transversal and between the parallels, they will add to  (that is, they will be supplementary).  Such angles are called same-side interior angles:

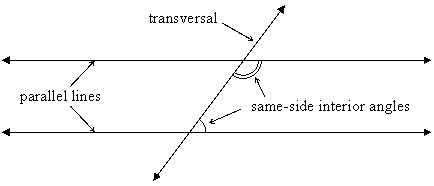
For his parallel postulate, Euclid used the contrapositive of this:  If two coplanar lines are intersected by a transversal and a pair of same-side interior angles do not add to , then they intersect on the side where the sum is less than .  You can see that this is a rather awkward statement and more difficult to understand than the parallel postulate we use today (which is sometimes called the Playfair Postulate, named after the dude who thought it up).

 

toc | return to top | next page