Arcs of Intersecting Chords
If two chords intersect, their vertical angles are congruent, and each vertical angle is the average of the two intercepted arcs:
 |
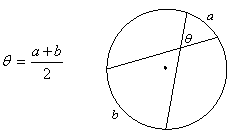We can prove this result by joining endpoints of the chords to form a triangle with θ as an exterior angle:
 |
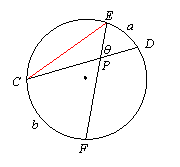θ is an exterior angle of triangle CEP, so it is the sum of the two remote interior angles, angles ECD and CEF. But these are inscribed angles with intercepted arcs whose measures are a and b, so each measures half its arc:
Content ©2009. All Rights Reserved.
Date last modified: March 26, 2009.
Created with SoftChalk LessonBuilder