Basic Terms

Circles

A circle is a set of points equidistance from a common point called its center.  We name different circles by specifying their centers:

Chords

A chord in a circle is a segment with its endpoints on the circle.  If a chord passes through the center of the circle, then it is also called a diameter of the circle.  (Sometimes the word diameter also refers to the length of the diameter.)

A radius of a circle is a segment with one endpoint on the circle and the other at the center of the circle.  (The word radius can also refer to the length of the radius.)

Secants and Tangents

A line that intersects a circle in two points of a circle is called a secant.  A secant is like an "extended chord."

A line that touches a circle at only one point is called a tangent, and the point is called the "point of tangency."

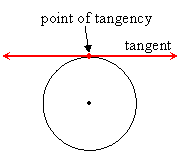Rays and segments are also said to be tangent to a circle if they are part of a tangent line.

Two circles are said to be tangent if they touch at exactly one point:

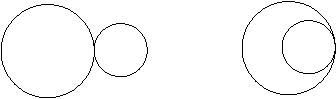Common Tangents

Example 1:  How many lines containing point P are tangent to the circle?

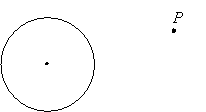Solution:  There are two tangent lines:

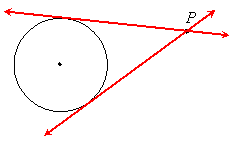A line that is tangent to two circles is called a "common tangent."

Tangents to Two Circles

Example 2:  How many common tangents do these two circles have?

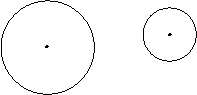Solution:  They have 4 common tangents:

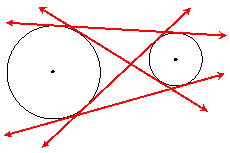Arcs and Central Angles

A central angle of a circle is an angle whose vertex is the center of the circle.  In the diagram, angle AOB is a central angle of the circle with center O:

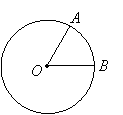If the measure of the angle is less than 180^o, then the points on the circle that are interior to the angle form a minor arc with endpoints A and B:

The other points on the circle form a major arc:

Naming arcs

Minor arcs are named by their endpoints with an arc over them, like this:

Major arcs are named using three points, where the middle point is any point on it other than the endpoints and the other two are the endpoints:

Return to Lesson 5